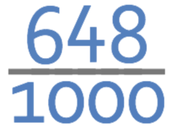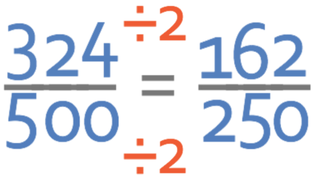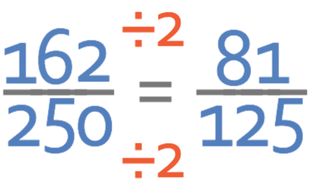1.1 B) Decimals
The place values in decimal numbers have specific names. Here are the place values:
The first place value after the decimal point for a number is known as the tenths. This is because a 1 in this place value means one tenth of a whole; if there were 10 tenths, we would have a whole.
The second place value after the decimal point is known as the hundredths and this is because a 1 in the hundredth place value would be one hundredth of a whole; it would take 100 hundredths to make a whole. A tenth is worth 10 hundredths.
The third place value after the decimal point is the thousandths, which is because a 1 in this place value would be 1 thousandths of a whole. A tenth is worth 100 thousandths and a hundredth is worth 10 thousandths.
It is very useful to know what each of the place values are in terms of another because from this information, we are able to convert a decimal into fractions.
Example 1
Convert the number below into a mixed number.
A mixed number is a number that contains an integer (a whole number) and a proper fraction (by proper fraction, we mean that the numerator of the fraction is smaller than the denominator).
The first step in answering this question is to place 78.65 in the grid above. The 78 will be the number in the mixed fraction and this is because there are 78 wholes in 78.65.
In 78.65, there are 65 hundredths. We can write the decimal part of the number as 65/100. Therefore, the number as a mixed fraction is:
The next step is to make sure that we simplify the fraction, which we do by dividing the numerator and the denominator of the fraction by the highest common factor between the numerator and the denominator. The highest common factor between 65 and 100 is 5. Therefore, we divide both the numerator and the denominator by 5. This results in the mixed number being:
Example 2
Convert the number below into a fraction:
The first place value after the decimal place is the tenths, the second place value is the hundredths and the third (and in this case final) place value the thousands. The number is shown in the table below.
In 0.648, there are 648 thousandths. Therefore, as a fraction, 0.648 is:
We now need to simplify our answer. We are able to go straight to the simplified answer by dividing both the numerator and the denominator by the highest common factor between the numerator and the denominator. However, it is fairly hard to see straight away what the highest common factor is between 648 and 1000. Therefore, as both of the numbers are even, they both have a factor of 2, so it would be a good start to divide the numerator and denominator by 2.
These two numbers are both even, so we can divide the numerator and the denominator by 2 again.
Again, these two numbers are even, so we can divide the numerator and the denominator by 2.
There are no common factors between 81 and 125, which means that 81/125 is 0.648 in its simplest form.