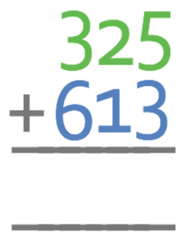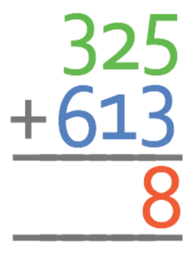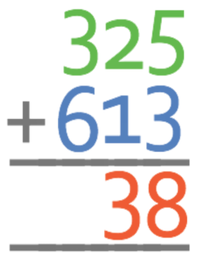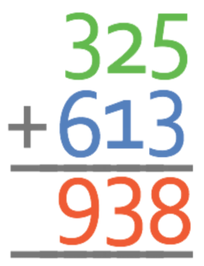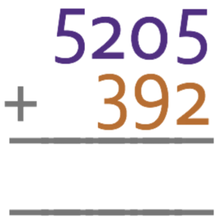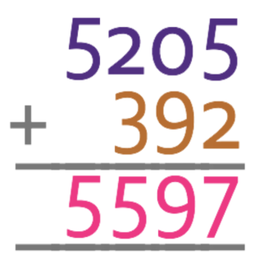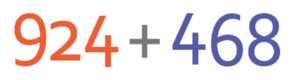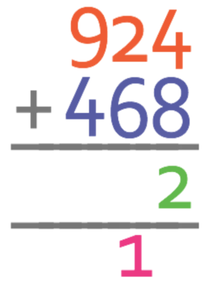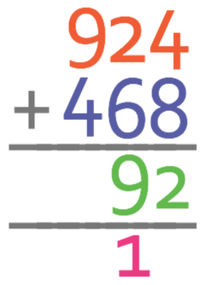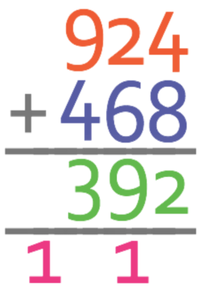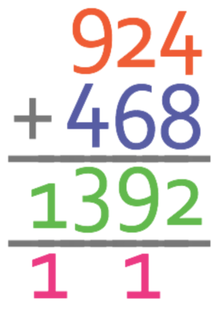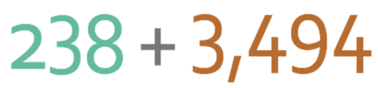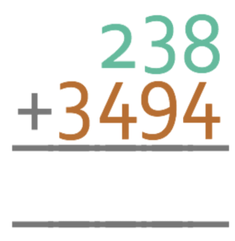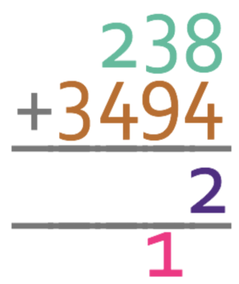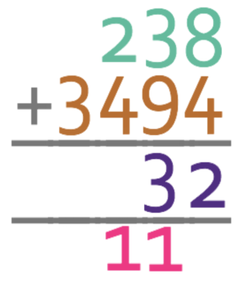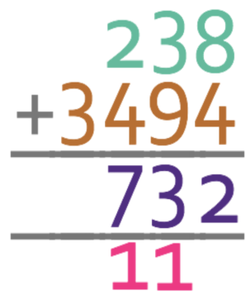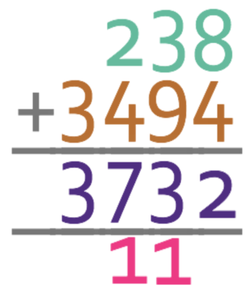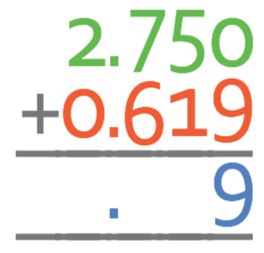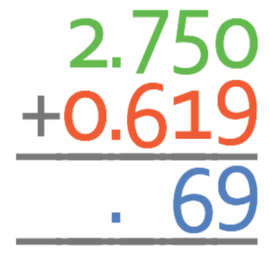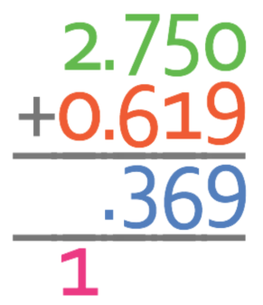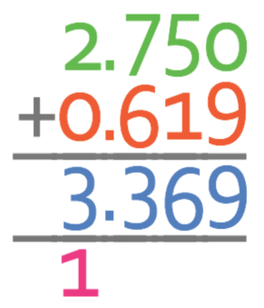Back to AQA Number (F) Home
1.1 D) Addition
1.1 D) Addition
The best method of addition when you are unable to use a calculator is column addition. Column addition is where you write the numbers that you are adding below one another with all of the place values lined up; we will have all of the units in a column, all of the tens in a column, all of the hundreds in a column etc. When you are undertaking column addition, you need to ensure that you lay out your columns correctly and cleanly.
Example 1
Complete the calculation below:
Complete the calculation below:
We are going to be using column addition to work out this question. We need to make sure that we line up all of the place values correctly. When writing out your number, you may find it easier to start written the units, then the tens, hundreds, etc, so that the place values line up.
We start by adding the units together; 5 + 3 = 8. 8 is less than 10, which means that we do not need to carry anything (we will be carrying in example 3). We write 8 for the units in our answer.
The next step is to add the numbers in the tens column, which are 2 (20) and 1 (10). These added together gives 3 (30) and as 3 is less than 10, we place 3 for the tens in our answer.
The final step is to add the hundreds. We add 3 (300) and 6 (600) together, which gives 9 (900). Therefore, we write 9 for the hundred in our answer.
The answer is 938.
Example 2
Complete the calculation below:
Complete the calculation below:
Like the previous example, the first step is to line all of the place values up correctly. It is easier to line the place values up by writing the numbers starting from the units.
We start by adding the units of the two numbers together, which gives us 7 (5 + 2). 7 is less than 10, which means that we do not need to carry anything.
We now add the numbers in the tens column, which gives us 9 (0 + 9).
We add the numbers in the hundred column, which gives us 5 (2 + 3).
The final step is to add the numbers in the thousands column. There is only one number in this column (there are no thousands in 392). This means that there will be a 5 thousands in the answer.
Therefore, the answer is 5,597.
Example 3 – Carrying
In both of the questions that we have looked at, there was no carrying. Carrying occurs whenever the sum of the numbers in the respective columns is greater than 9. Whenever we need to carry, we place the number in the tens place value underneath the next column. This will make more sense after we have gone through a few examples.
Compete the calculation below:
In both of the questions that we have looked at, there was no carrying. Carrying occurs whenever the sum of the numbers in the respective columns is greater than 9. Whenever we need to carry, we place the number in the tens place value underneath the next column. This will make more sense after we have gone through a few examples.
Compete the calculation below:
The first step in answering this question is to line all of the place values up.
We then start by adding the numbers in the units column, which gives us 12. 12 is greater than 10, which means that we write the 2 in the units column for the answer and carry the 1 (the 10) into the next column. The working is shown below.
We now add all of the numbers in the tens column. When we do this, we need to make sure that we add the carried 1 from the units column. Therefore, we add 2, 6 and 1, which gives us 9.
We now add the numbers that are in the hundred column and this gives us 13 (9 + 4). 13 is greater than 9, so we need to carry the 1 in 13.
The final step is to add the thousands. There is only one number in the thousands column and this has come from the carried number when adding the hundreds. This means that we place a 1 in the thousand column.
The answer for this calculation is 1,392.
Example 4 – Carrying
Complete the calculation below:
Complete the calculation below:
Like the previous examples, the first step is to line the place values up.
We start by adding the units in the two numbers; we add 8 and 4 together, which gives us 12. 12 is greater than 9, which means that we carry the 1 in 12 to the next place value column (the tens).
We now add the numbers in the tens column together with the number that we have carried; 3 + 9 + 1 (the carried 1), which gives us 13. 13 is greater than 9 and this means that we carry the 1 in the 13 to the next place value column (the hundreds).
We now add the hundreds; 2 + 4 + 1 (the carried 1), which gives us 7. 7 is less than 9, thus meaning that there is nothing to carry.
We now add the numbers in the thousands column. There are no thousands 238, 3 thousands in 3,494 and no thousands to carry. Therefore, there will be a 3 in the thousands column in the answer.
Therefore, the answer to this question is 3,732.
Example 5 – Decimals
Complete the calculation below:
Complete the calculation below:
The first step is to line up the place values.
When we line the number up, we can see that the top number does not have any thousandths. Therefore, we can write a 0 in the thousandth for this number. Also, it is worth putting the decimal point down for the answer straight away so that we do not forget it.
We then start by adding the numbers in the lowest place values, which for these numbers is the thousandths; we add 0 to 9, which gives 9.
We now move onto the next place value, which is the hundredths; we add 5 to 1, which gives 6.
We now move onto adding the numbers in the tenths column, which gives 13 (7 + 6). 13 is greater than 9 and this means that we carry the 1 into the next place value, which is the units.
We now add the units; we add the 2, 0 and the carried 1. This gives 3.
Therefore, the answer to this calculation is 3.369.
End Note
The key thing to remember with column addition is to make sure that you carefully line up all of the place values in each of the numbers. You then start adding from the smallest place values.
If you are asked to add 3 numbers together, you would start in exactly the same way as we have for these questions; you would line all of the place values up for the 3 numbers. You would then add from the smallest place values and continue the exact same process as the questions above. Alternatively, you could add two of the numbers together, and then add the result to the final number.
The key thing to remember with column addition is to make sure that you carefully line up all of the place values in each of the numbers. You then start adding from the smallest place values.
If you are asked to add 3 numbers together, you would start in exactly the same way as we have for these questions; you would line all of the place values up for the 3 numbers. You would then add from the smallest place values and continue the exact same process as the questions above. Alternatively, you could add two of the numbers together, and then add the result to the final number.