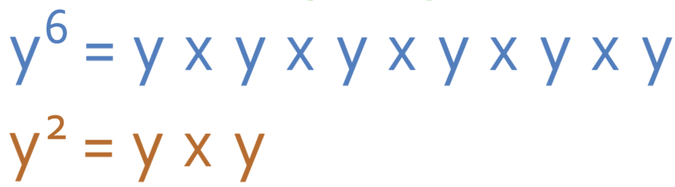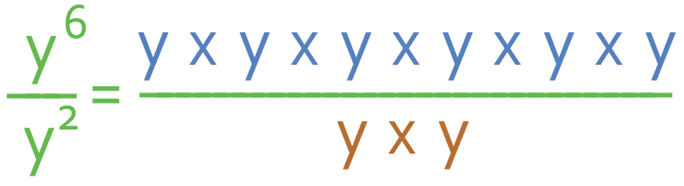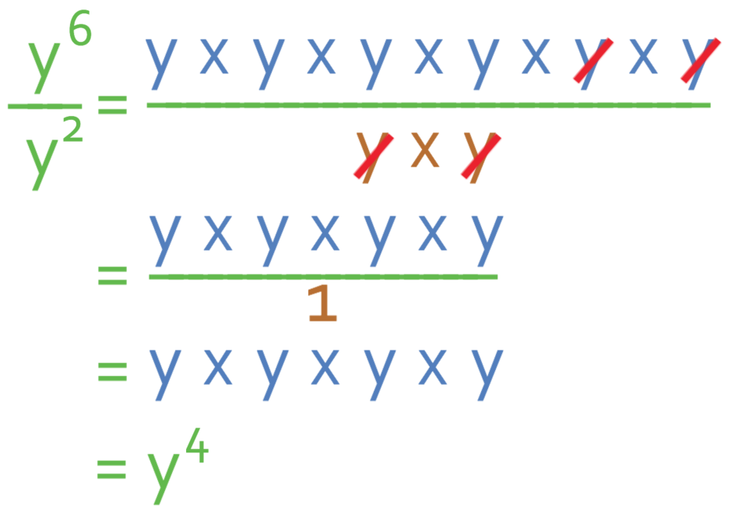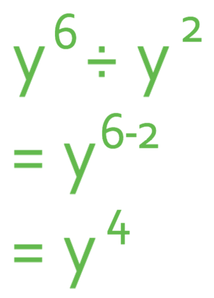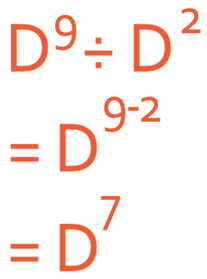1.6 C) Division
When we are dividing powers that have the same base, we take the powers away from each other.
Example 1
Simplify:
Like with multiplication, I am going to write out both of these terms in full. This gives us:
I am going to covert the calculation into a fraction and sub each of the terms in full into their appropriate place in the fraction.
We can simplify this fraction because the numerator and the denominator both have y x y (or y2) in common. This leaves the fraction as:
Therefore, the answer to this question is y4.
Now let’s answer the question using the rule. The bases are the same, which means that we can take the powers away from one another.
This gives us the same answer that we got before when we were writing each of the terms out in full.
The writing of each of the terms out in full was there to show you where the rule comes from/ to prove that the rule works. From now on you can just use the rule, which is that we when we divide powers with the same base, we take the powers.
Example 2
What is:
The bases are the same, which means that we can take the powers away from one another.
The answer is D7.
As with multiplication, we need to make sure that the bases of the powers are the same because if they are not the same, we will be unable to divide/ combine the indices. For example, we would be unable to combine the indices A7 ÷ B6 because the bases are different; one of the bases is A and the other base is B.
We follow the rule for division, even if we have a negative power. We will be looking at what a negative power means later on in this whole indices section.