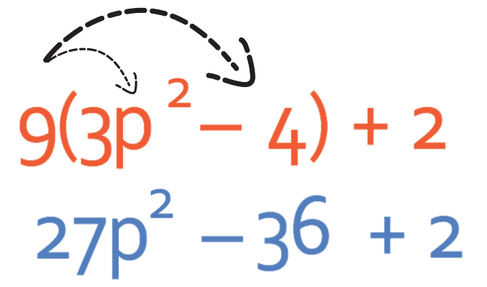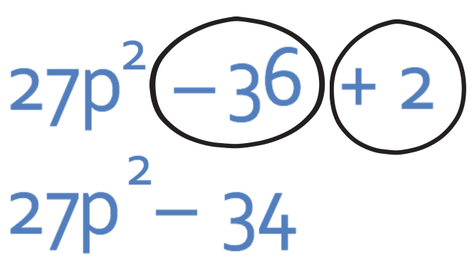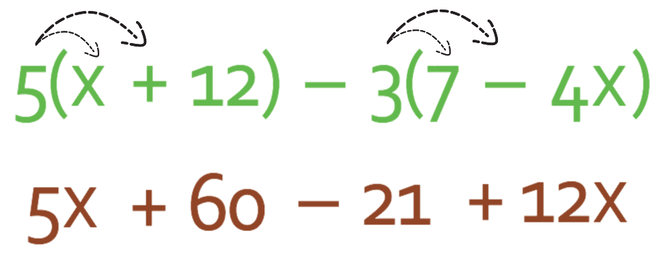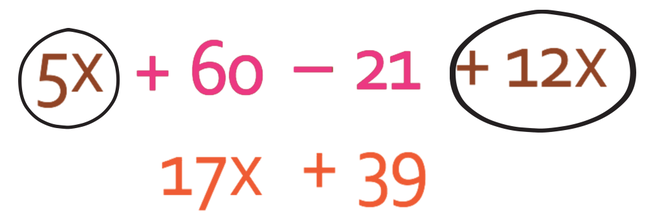Back to AQA Algebra Introduction (F) Home
2.1 G) Expand & Simplify
2.1 G) Expand & Simplify
Whenever we do anything in maths, we need to make sure that the answer is given in it’s simplest form (we collect like terms, simplify fractions etc.). In this section, we are going to have two examples which require you to simplify your answer.
Example 1
Expand and simplify the expression below.
Expand and simplify the expression below.
Another rule in maths is that we must always remember BIDMAS or BODMAS (Brackets, Indices or Other, Division, Multiplication, Addition and Subtraction). Click here to be taken through to the section that discusses BIDMAS and BODMAS in more detail. Therefore, the first step in answering this question is to multiply out the brackets. The expression becomes:
We now need to check whether the expression can be simplified. This equation can be simplified because there are two numbers in the expression; -36 and +2. We can combine these two numbers to give -34. The simplified expanded out expressions becomes.
Example 2
Expand the expression below making sure that your answer is in its simplest form.
Expand the expression below making sure that your answer is in its simplest form.
With the expression above, we need to be very careful when we are multiplying out the second bracket. This is because the number on the outside of the second bracket is -3, which means that we need to multiply the two terms inside the bracket by -3.
Let’s multiply out the first bracket and the second bracket separately. The expanded first bracket is:
Let’s multiply out the first bracket and the second bracket separately. The expanded first bracket is:
When we multiply -3 by 7, we obtain -21 (a negative multiplied by a positive is a negative, and 3 multiplied by 7 is 21. These two combined gives us -21). For the second term, we are multiplying -3 by -4x. The expanded term will be positive because two negatives multiplied together gives a positive. We then multiply the numbers; 3 multiplied by 4, which gives us 12. The final step is to multiply the unknowns. There are no x’s in -3, which means that the second term will have an x in it. Therefore, the second term in the second expanded bracket is 12x.
There are two terms that have x in them and two terms that are numbers. This means that we are able to simplify the above expression. Whenever simplifying expressions, you may find it easier to highlight or circle the different types of terms that are in the expression. When you are highlighting the different terms, you need to make sure that you include the sign that appears before the term. In the expression below, I have circled the x terms and change the font colour for the terms that only contain numbers.
The expression above is now in its simplest form.