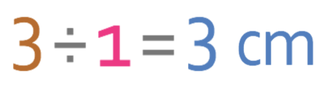Back to AQA Loci & Construction (F) Home
4.2 A) Loci
4.2 A) Loci
Before we start working through the content, it is worth mentioning that loci is the plural and locus is the singular.
A locus is a path formed by a point that moves according to a rule (the rules can vary greatly).
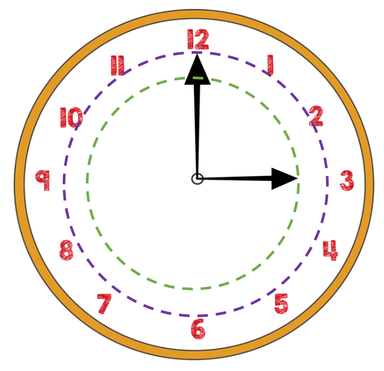
A common locus is around a fixed point. We are going to use the example of the hands on a clock. The tip of the hands of the clock are always going to be the same distance away from the centre of the clock; this is known as being equidistance. There are two different loci on the diagram below; one of the loci is for the minute hand and the other is for the hour hand.
A locus is a path formed by a point that moves according to a rule (the rules can vary greatly).
A common locus is around a fixed point. We are going to use the example of the hands on a clock. The tip of the hands of the clock are always going to be the same distance away from the centre of the clock; this is known as being equidistance. There are two different loci on the diagram below; one of the loci is for the minute hand and the other is for the hour hand.
Example 1
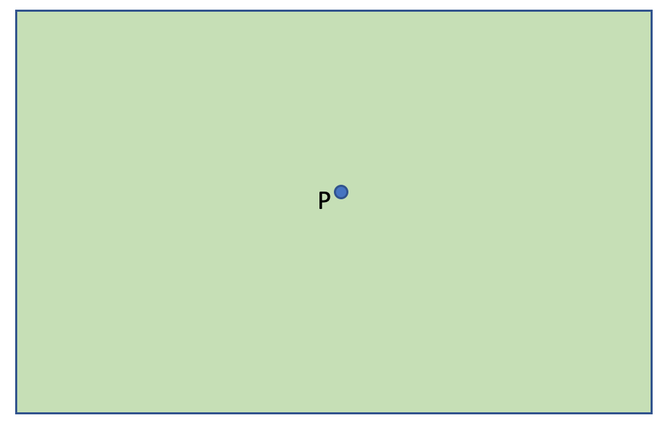
We are now going to have an example whereby we are going to draw a locus. We are going to pretend that we have a horse that is tied up to a post (the post is labelled P on the diagram below). The horse is attached to a 5 m long rope. What is the locus of the horse if it walks around the post at full extension? Use the scale of 1 cm = 1 metre. Click here for a printable version.
We are now going to have an example whereby we are going to draw a locus. We are going to pretend that we have a horse that is tied up to a post (the post is labelled P on the diagram below). The horse is attached to a 5 m long rope. What is the locus of the horse if it walks around the post at full extension? Use the scale of 1 cm = 1 metre. Click here for a printable version.
The first step is to find out the length of the rope on the diagram. We are able to do this by dividing the length of the actual rope by the scale that we are given. The length of the rope is 5 metres and the scale on the diagram is 1 cm is 1 metre. Therefore, the calculation for finding the length of the rope is:
The length of the rope on our diagram is 5 cm.
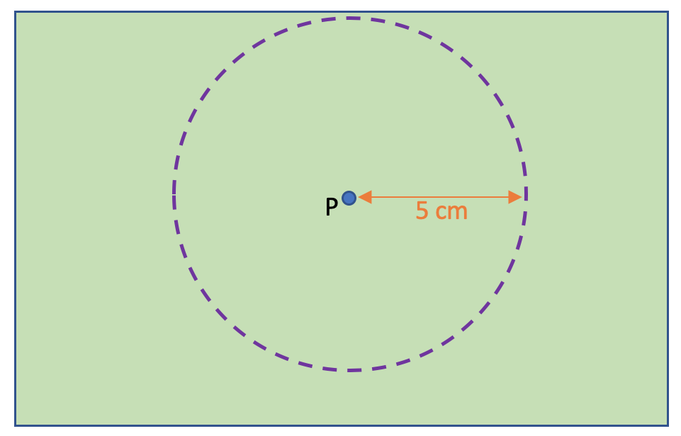
We are going to be using a compass to draw the locus. We set the distance between the point and the pencil on the compass to 5 cm, which is the length of the rope according to our scale. We use a ruler to set the correct length for the compass.
When the length of the compass is set correctly, we place the point of the compass at the centre of the post and gentle move the pencil part of the compass round. This will create a circle around the post, which will be the locus for this example.
We are going to be using a compass to draw the locus. We set the distance between the point and the pencil on the compass to 5 cm, which is the length of the rope according to our scale. We use a ruler to set the correct length for the compass.
When the length of the compass is set correctly, we place the point of the compass at the centre of the post and gentle move the pencil part of the compass round. This will create a circle around the post, which will be the locus for this example.
Example 2
The rules for loci can vary greatly. Both the clock and horse example have had loci around a particular point. We are now going to have a look at loci along a straight line.
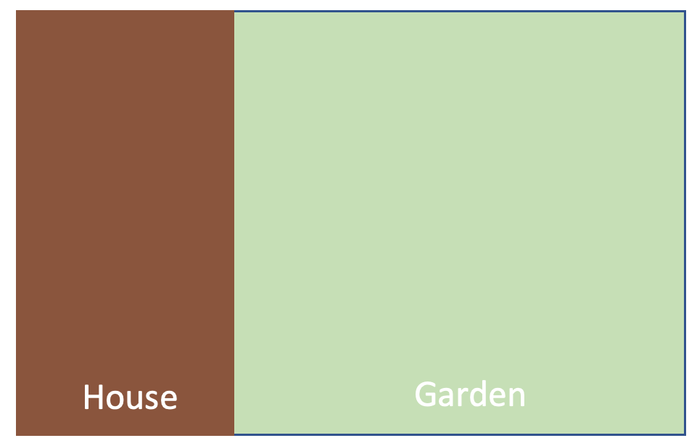
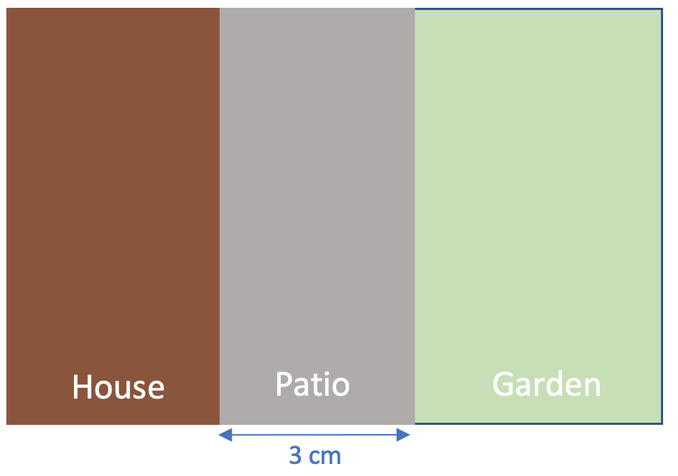
I am going to create a patio on the outside of my house. I want the edge of the patio to be 3m from the outside of my house. The diagram below shows my house and my garden. Draw the patio on the diagram below and use the scale 1 cm = 1 metre.
The rules for loci can vary greatly. Both the clock and horse example have had loci around a particular point. We are now going to have a look at loci along a straight line.
I am going to create a patio on the outside of my house. I want the edge of the patio to be 3m from the outside of my house. The diagram below shows my house and my garden. Draw the patio on the diagram below and use the scale 1 cm = 1 metre.
Like the example before, the first step is to use the scale to find the distance of the patio on the diagram. We find the distance on the diagram by dividing the actual distance by the scale. The actual distance is 3 metres and the scale is 1 cm is 1 m. Therefore, we undertake the following calculation:
The distance of the patio on the diagram will be 3 cm.
The outside of my house is a straight line. We draw the end of the patio by measuring 3 cm from each end of the house; 3 cm from the top and 3 cm from the bottom. We then draw a line that connects these points.
The outside of my house is a straight line. We draw the end of the patio by measuring 3 cm from each end of the house; 3 cm from the top and 3 cm from the bottom. We then draw a line that connects these points.
Example 3
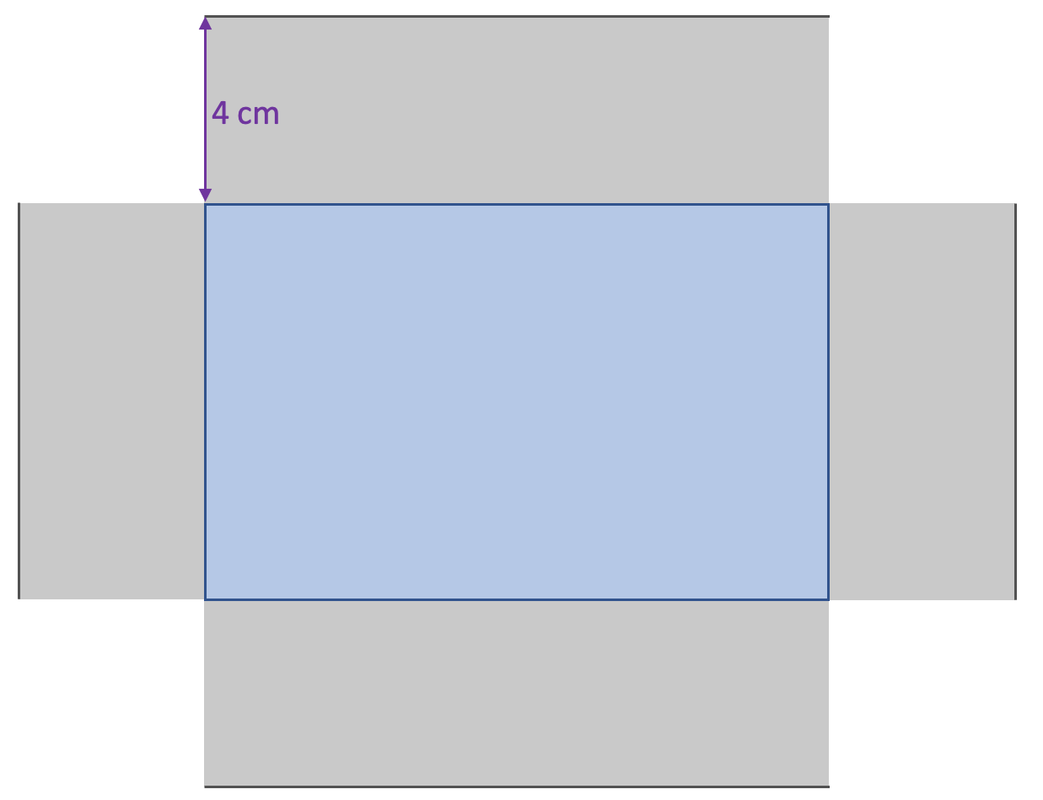
I have a rectangular swimming pool. There is a diagram of my pool below with the scale 1 cm is 1 metre. I want to build a path around the edge of the swimming pool. The width of the path must be 4 metres.
I have a rectangular swimming pool. There is a diagram of my pool below with the scale 1 cm is 1 metre. I want to build a path around the edge of the swimming pool. The width of the path must be 4 metres.
The first step is to work out the scale. We are told that the path is going to be 4 m away from the edge of the pool. The scale for the diagram is 1 cm is 1 m. Therefore, the width of the path of the diagram is 4 cm (4 ÷ 1 = 4).
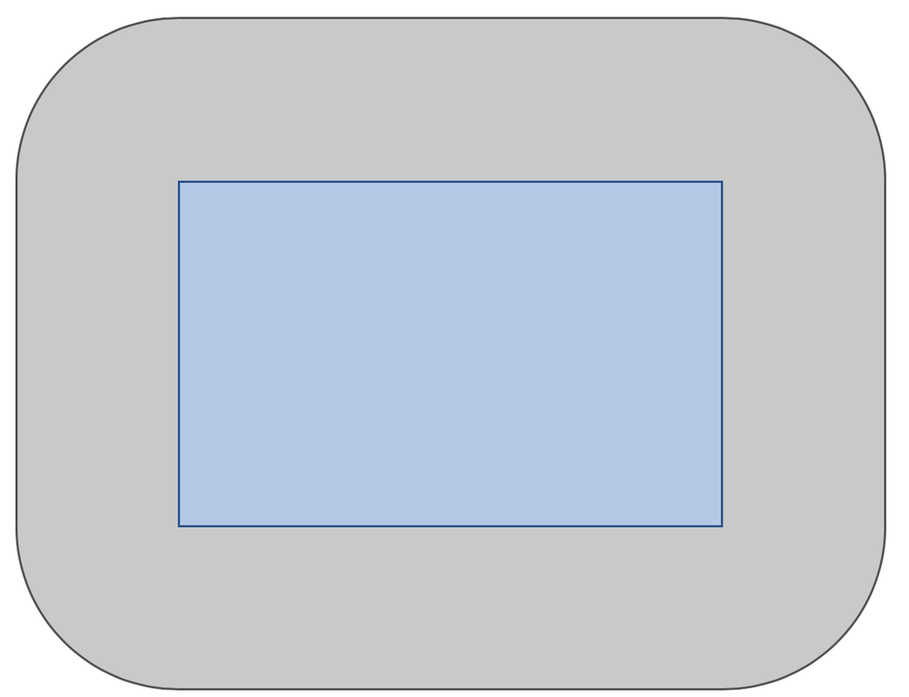
I am going to split the pool into two separately groups; the straight sides and the corners. I am going to create the locus for the straight lines first. I do this by going to the end of each of the straight lines and measuring 4 cm out. I then connect the points for each line. This is exactly the same as the process patio example.
I am going to split the pool into two separately groups; the straight sides and the corners. I am going to create the locus for the straight lines first. I do this by going to the end of each of the straight lines and measuring 4 cm out. I then connect the points for each line. This is exactly the same as the process patio example.
The next step is to draw the locus for the corners. We use a compass to draw a locus around a point. We do this by setting the compass to 4 cm. We then place the point of the compass on each of the corners and draw a quarter of a circle. We obtain what is show in the diagram below.