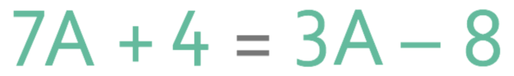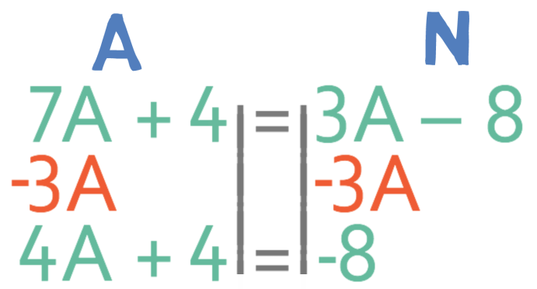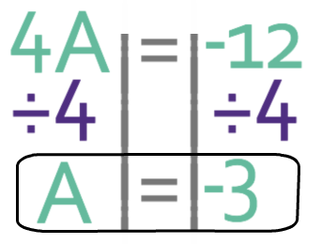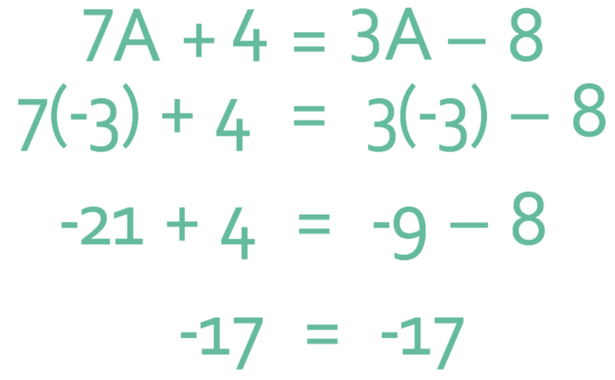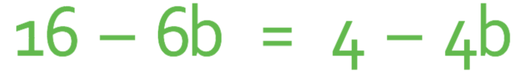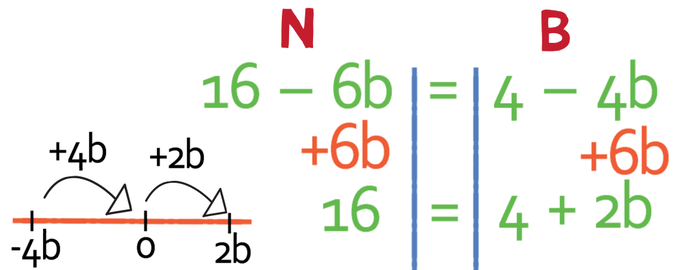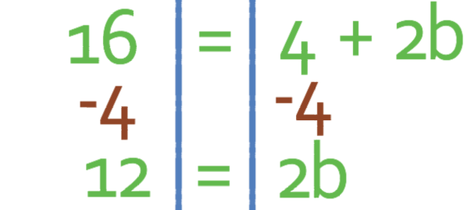Back to AQA Linear Equations (H) Home
2.2 C) Linear Equations: Unknowns and Numbers on Both Sides
2.2 C) Linear Equations: Unknowns and Numbers on Both Sides
If we are given a question with unknowns on both sides, we must get all of the unknowns to one side and all of the numbers to the other side. We then divide both sides by the coefficient of the unknown.
Example 1
Find the value of A.
Find the value of A.
It is easier to work with positive values of the unknown, therefore we get all of the unknowns to the side with the greatest number of unknowns. This means that we are going to get all of the unknowns to the left side of the equation because 7A is greater than 3A. Therefore, we want to move the 3A from the right side to the left, which we are able to do by doing the opposite; we take 3A from both sides.
The next step is to get all of the numbers on the right side, which means that we need to move the 4 that is currently on the left side to the right. We are able to do this by doing the opposite; we take 4 from both sides of the equation.
We want to know what A is and not what 4A is. Therefore, we divide both sides by 4 (the coefficient of the unknown that we are looking for).
This tells us that A is equal to -3.
We can check that we have the correct value for A by subbing A in as -3 into the equation that we were given at the beginning (we should always use the equation at the beginning rather than any manipulated equation because we may have made a mistake whilst manipulating the equation and this would mean that we are checking an incorrect equation).
We can check that we have the correct value for A by subbing A in as -3 into the equation that we were given at the beginning (we should always use the equation at the beginning rather than any manipulated equation because we may have made a mistake whilst manipulating the equation and this would mean that we are checking an incorrect equation).
This equation works, which means that we have the correct value for A; A is -3.
Example 2
Find the value of b.
Find the value of b.
We are going to answer this question by getting all of the unknowns to one side and all of the numbers to the other side. We get the unknowns to the side that has the greatest number of unknowns, which is the left side because -4b is greater than -6b. Therefore, we want to have all of the unknowns on the right and all of the numbers on the left. This means that we need to move the -6b from the left to the right. We do this by doing the opposite, which is to add 6b to both sides of the equation.
We now need to move the 4 that is currently on the right side to the left side. We do this by taking 4 from both sides.
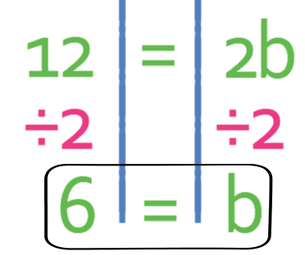
We want to find the value of b and not 2b. Therefore, we divide both sides of the equation by 2.
This tells us that b is 6.
We are able to check that we have found the correct value for b by subbing in b as 6 into the first equation (the equation at the top).
We are able to check that we have found the correct value for b by subbing in b as 6 into the first equation (the equation at the top).
This equation works, which means that we have found the correct value for b; b is 6.