Back to AQA Percentages (H) Home
3.2 I) Increasing or Decreasing by a Certain Percentage – Calculator: Part 2
3.2 I) Increasing or Decreasing by a Certain Percentage – Calculator: Part 2
In this section, we are going to have a look at a few worded examples. The content in this section builds on the content that was discussed in the previous section. Therefore, make sure that you have worked through the previous section before working through this one (click here to be taken through to the previous section).
Example 1
I purchase an antique clock for £600. Since purchasing the clock, the value of the clock has increased by 27%. What is the clock now worth? Use the multiplier method.
We are able to find the value of the clock by multiplying the multiplier by the what I purchased the clock for. The calculation is shown below:
I purchase an antique clock for £600. Since purchasing the clock, the value of the clock has increased by 27%. What is the clock now worth? Use the multiplier method.
We are able to find the value of the clock by multiplying the multiplier by the what I purchased the clock for. The calculation is shown below:
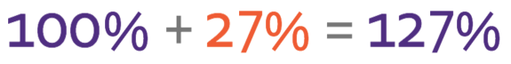
The question tells us that the purchase price is £600. Currently, we do not have the value for the multiplier by we can work it out from the information given in the question. We are told that the value of the clock has increased by 27%. Therefore, the first step in finding the multiplier is to add 27% to 100%.
We turn this into a multiplier by dividing by 100.
We now have the multiplier (1.27) and the purchase value of the clock (£600), which means that we work out what the clock is worth now.
The clock is now worth £762.
Example 2
I purchased a new car for £25,000. The value of the car decreases by 14.75% in the first year. What is the value of the car after the first year? Use the multiplier method to obtain your answer.
We find the value of the car by using pretty much the same formula as the previous question; we multiply the multiplier by the original value of the car.
I purchased a new car for £25,000. The value of the car decreases by 14.75% in the first year. What is the value of the car after the first year? Use the multiplier method to obtain your answer.
We find the value of the car by using pretty much the same formula as the previous question; we multiply the multiplier by the original value of the car.
We are told in the question that the original value of the car is £25,000. Currently, we do not have the multiplier value, but we are able to work it out from the information given in the question. The question tells us that the value of the car decreases by 14.75% in the first year. As the value of the car is decreasing, we take this percentage off of 100%.
We turn this into a multiplier by dividing by 100.
The multiplier value if 0.8525.
We now have the multiplier (0.8525) and the original value of the car (£25,000), which means that we can work out the value of the car after the first year.
We now have the multiplier (0.8525) and the original value of the car (£25,000), which means that we can work out the value of the car after the first year.
We are working with money, and this means that we need to make sure that our answer has 2 decimal places; we need to remember the 0 in the second decimal place (it may be the case that you will lose a mark if you wrote £21,312.5).
The value of the car after the first year is £21,312.50.
The value of the car after the first year is £21,312.50.