Back to AQA Probability (H) Home
Probability: Quiz 11 - Answers
Probability: Quiz 11 - Answers
1)
a) 40
b) 23
c) 13
d) 17
e) 5
f) 12
g) 8
h) 27
i) 25
j) 1/8
k) 3/8
l) 13/40
m) 17/40
n) 1/5
o) 5/8
2)
a)
b) 7/15
c) 1/3
d) 1/3
e) 2/3
f) 2/15
g) 1/5
h) 1/3
3)
b) 2/3
c) 1/2
d) 11/30
e) 3/10
f) 1/2
g) 11/15
h) 9/20
i) 11/24
4)
a)
b) 1/6
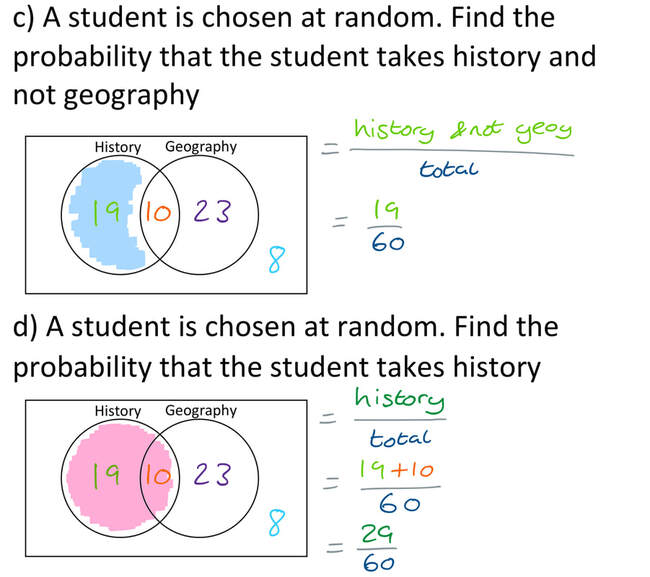
c) 19/60
d) 29/60
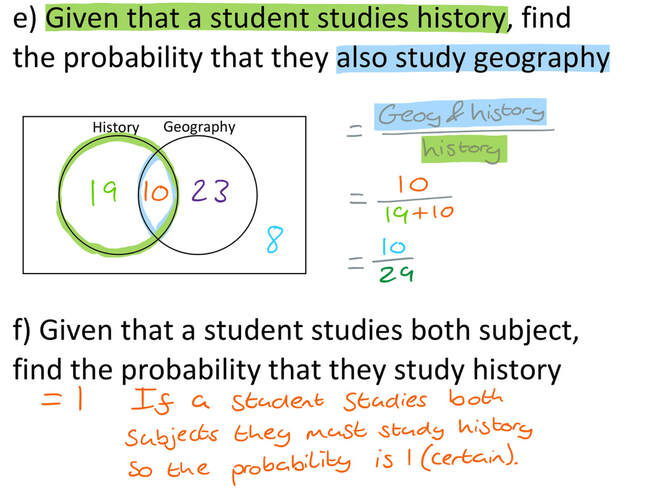
e) 10/29
f) 1
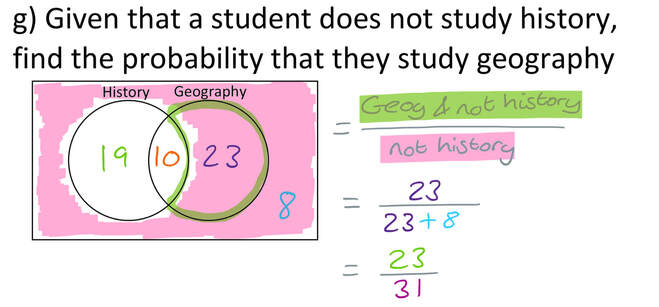
g) 23/31
5)
a)
b) 13/50
c) 19/50
d) 1/2
e) 13/45
6)
a)
b) 1/30
c) 2/5
d) 1/2
e) 1/2
f) 1/10
g) 1/12
h) 1/15
i) 3/20
j) 37/60
k) 17/20
l) 5/24
m) 6/13
n) 1/2
o) 5/8
p) 6/13
Working
Questions
Click here for a printout of the diagrams in this quiz.
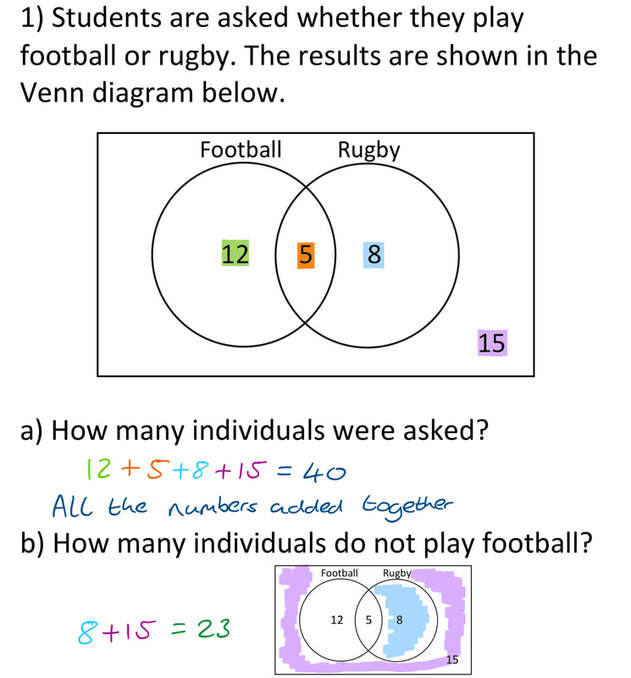
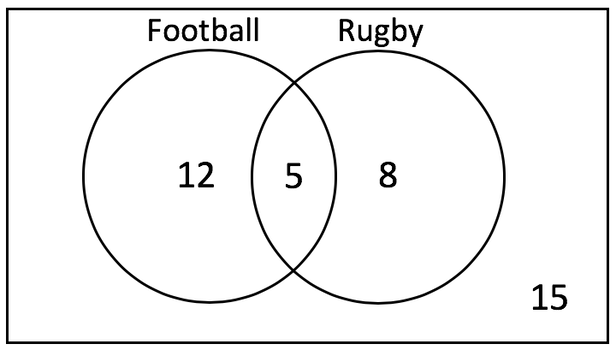
1) Students are asked whether they play football or rugby. The results are shown in the Venn diagram below.
Click here for a printout of the diagrams in this quiz.
1) Students are asked whether they play football or rugby. The results are shown in the Venn diagram below.
a) How many individuals were asked?
b) How many individuals do not play football?
c) How many individuals play rugby?
d) How many individuals play football?
e) How many individuals play rugby and football?
f) How many individuals play football and not rugby?
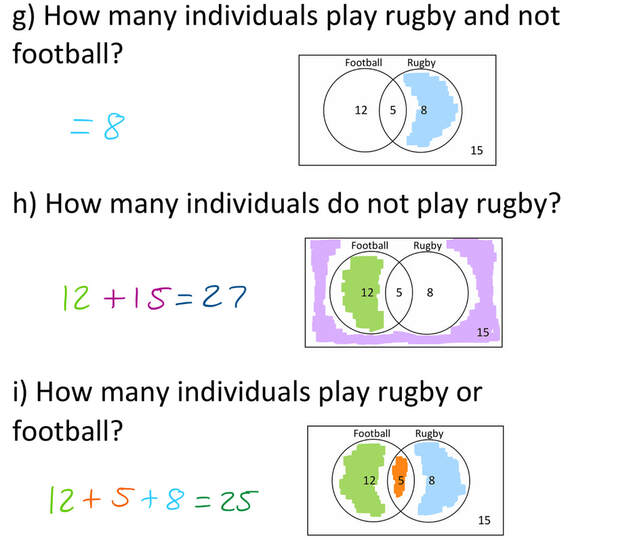
g) How many individuals play rugby and not football?
h) How many individuals do not play rugby?
i) How many individuals play rugby or football?
Find the probability of the following events occurring.
j) Choosing an individual that plays rugby and football
k) Choosing an individual that does not play rugby and does not play football
l) Choosing an individual that plays rugby
m) Choosing an individual that plays football
n) Choosing an individual that plays rugby and not football
o) Choosing an individual that plays rugby or football
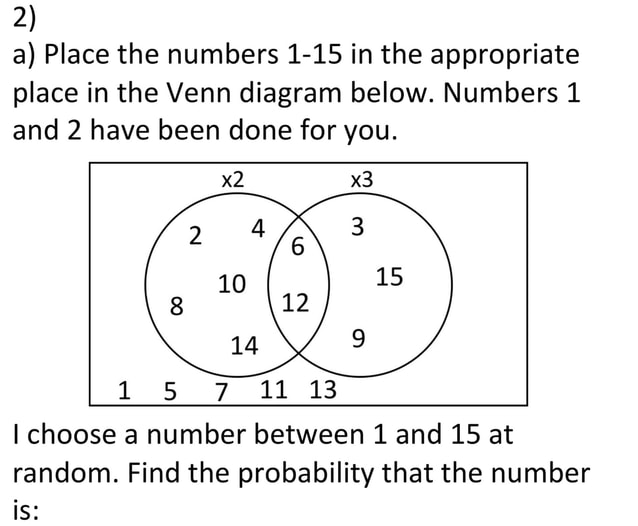
2)
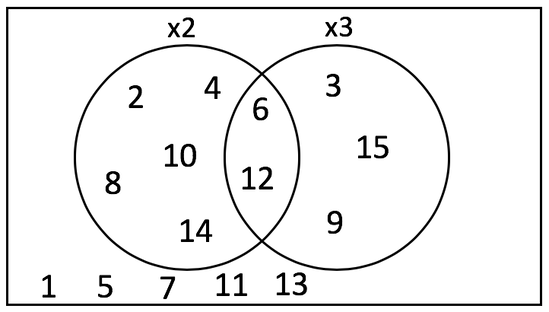
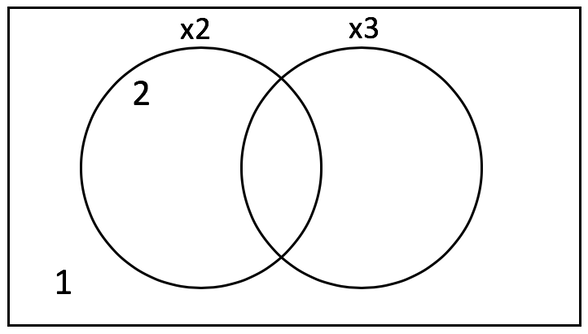
a) Place the numbers 1-15 in the appropriate place in the Venn diagram below. Numbers 1 and 2 have been done for you.
b) How many individuals do not play football?
c) How many individuals play rugby?
d) How many individuals play football?
e) How many individuals play rugby and football?
f) How many individuals play football and not rugby?
g) How many individuals play rugby and not football?
h) How many individuals do not play rugby?
i) How many individuals play rugby or football?
Find the probability of the following events occurring.
j) Choosing an individual that plays rugby and football
k) Choosing an individual that does not play rugby and does not play football
l) Choosing an individual that plays rugby
m) Choosing an individual that plays football
n) Choosing an individual that plays rugby and not football
o) Choosing an individual that plays rugby or football
2)
a) Place the numbers 1-15 in the appropriate place in the Venn diagram below. Numbers 1 and 2 have been done for you.
I randomly select a number between 1 and 15. Find the probability that the number is:
b) A multiple of 2
c) A multiple of 3
d) Not a multiple of 2 or 3
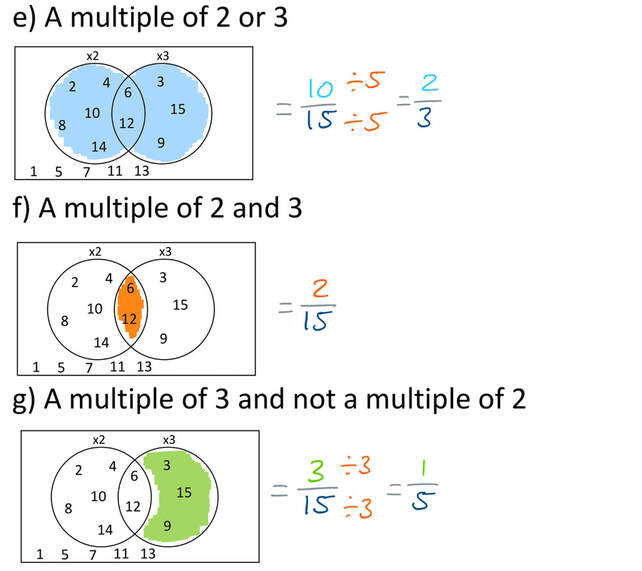
e) A multiple of 2 or 3
f) A multiple of 2 and 3
g) A multiple of 3 and not a multiple of 2
h) A multiple of 2 and not a multiple of 3
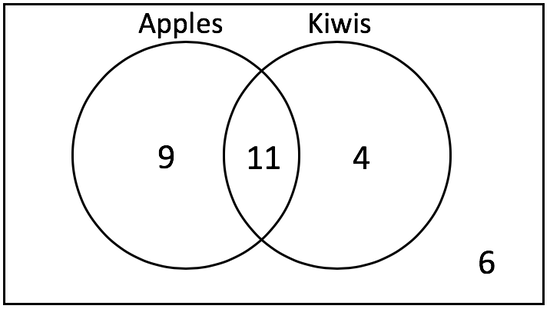
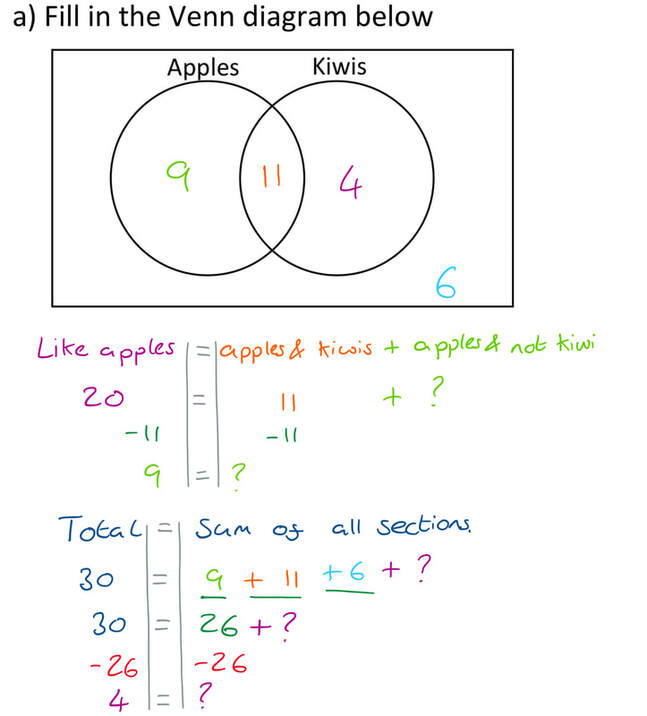
3) I ask 30 people whether they like apples and Kiwi. The outcomes were:
a) Fill in the Venn diagram below
b) A multiple of 2
c) A multiple of 3
d) Not a multiple of 2 or 3
e) A multiple of 2 or 3
f) A multiple of 2 and 3
g) A multiple of 3 and not a multiple of 2
h) A multiple of 2 and not a multiple of 3
3) I ask 30 people whether they like apples and Kiwi. The outcomes were:
- 11 people like both apples and kiwis
- 20 people liked apples
- 6 people did not like apples and also did not like kiwis
a) Fill in the Venn diagram below
Find the probability of the following events occurring:
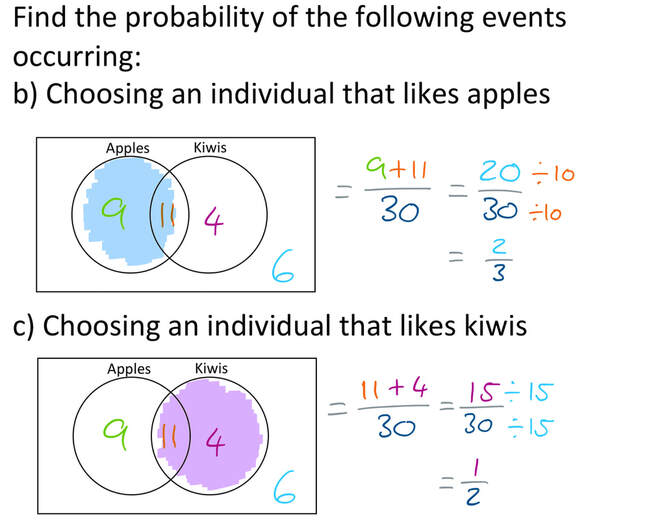
b) Choosing an individual that likes apples
c) Choosing an individual that likes kiwis
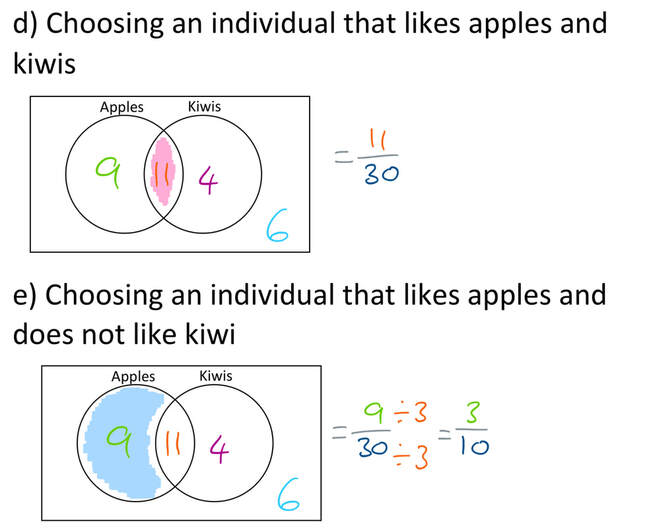
d) Choosing an individual that likes apples and kiwis
e) Choosing an individual that likes apples and does not like kiwis
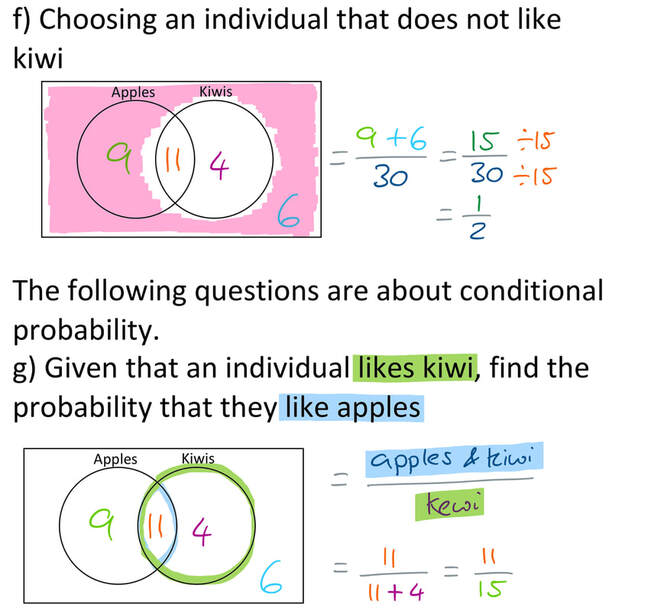
f) Choosing an individual that does not like kiwis
The following questions are about conditional probability.
g) Given that an individual likes kiwis, find the probability that they like apples
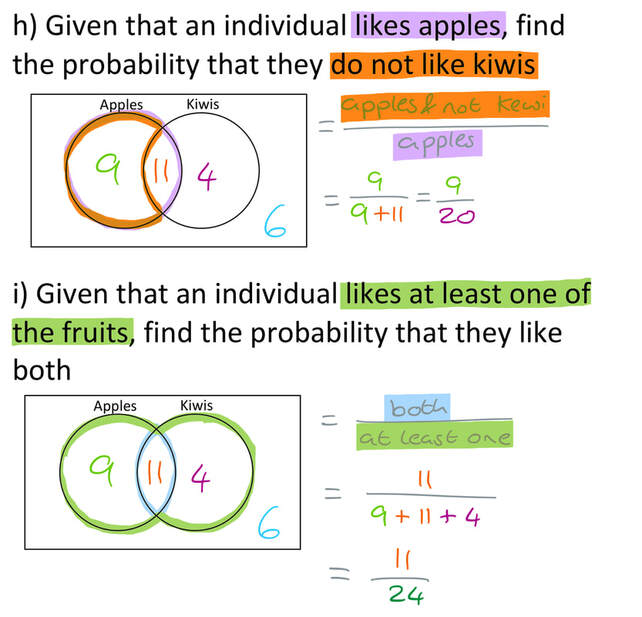
h) Given that an individual likes apples, find the probability that they do not like kiwis
i) Given that an individual likes at least one of the fruits, find the probability that they like both
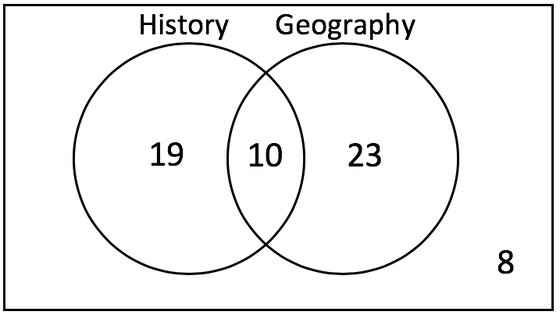
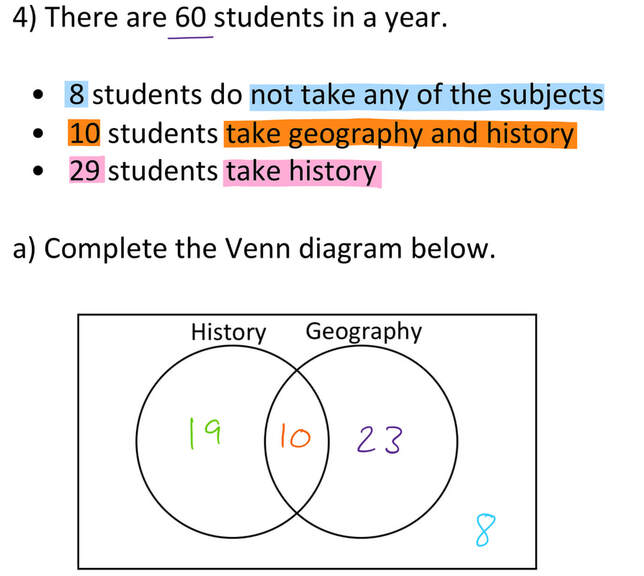
4) There are 60 students in a year.
a) Complete the Venn diagram below.
b) Choosing an individual that likes apples
c) Choosing an individual that likes kiwis
d) Choosing an individual that likes apples and kiwis
e) Choosing an individual that likes apples and does not like kiwis
f) Choosing an individual that does not like kiwis
The following questions are about conditional probability.
g) Given that an individual likes kiwis, find the probability that they like apples
h) Given that an individual likes apples, find the probability that they do not like kiwis
i) Given that an individual likes at least one of the fruits, find the probability that they like both
4) There are 60 students in a year.
- 8 students do not take any of the subjects
- 10 students take geography and history
- 29 students take history
a) Complete the Venn diagram below.
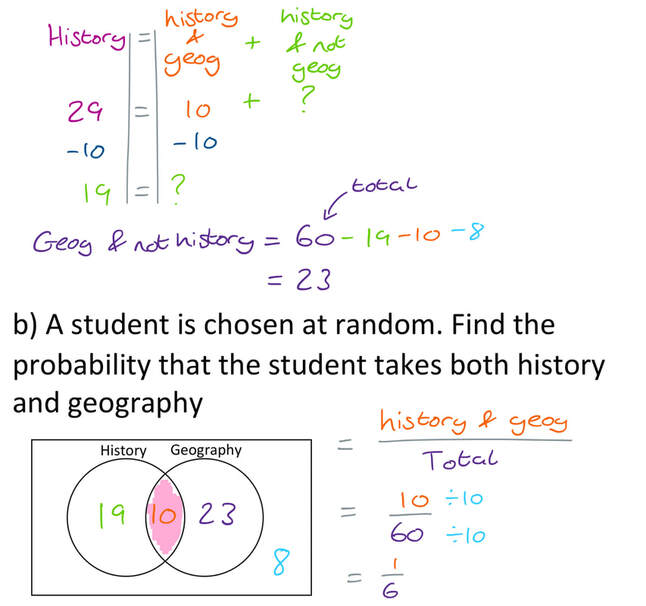
b) A student is chosen at random. Find the probability that the student takes both history and geography
c) A student is chosen at random. Find the probability that the student takes history and not geography
d) A student is chosen at random. Find the probability that the student takes history
e) Given that a student studies history, find the probability that they also study geography
f) Given that a student studies both subjects, find the probability that they study history
g) Given that a student does not study history, find the probability that they study geography
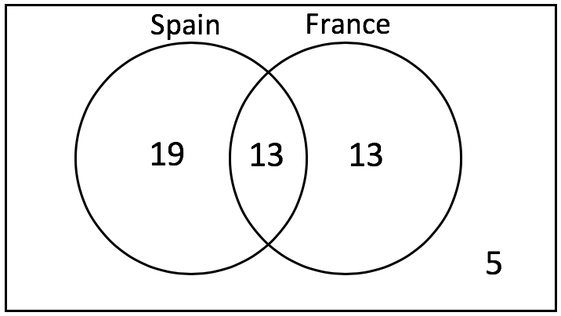
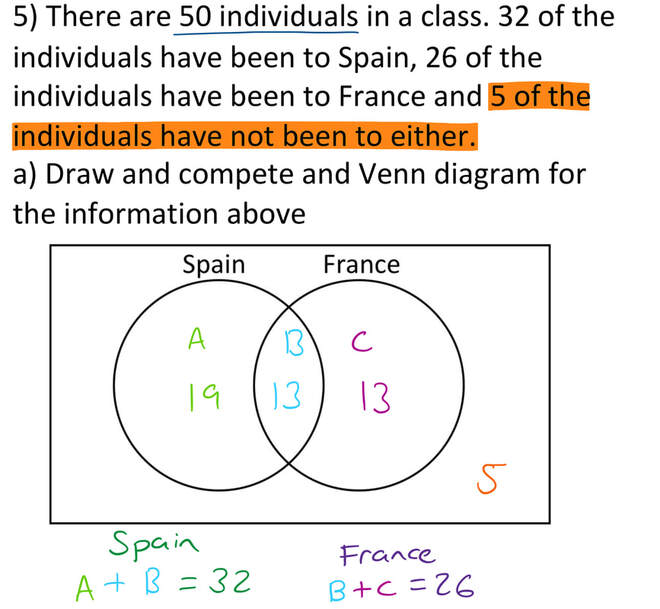
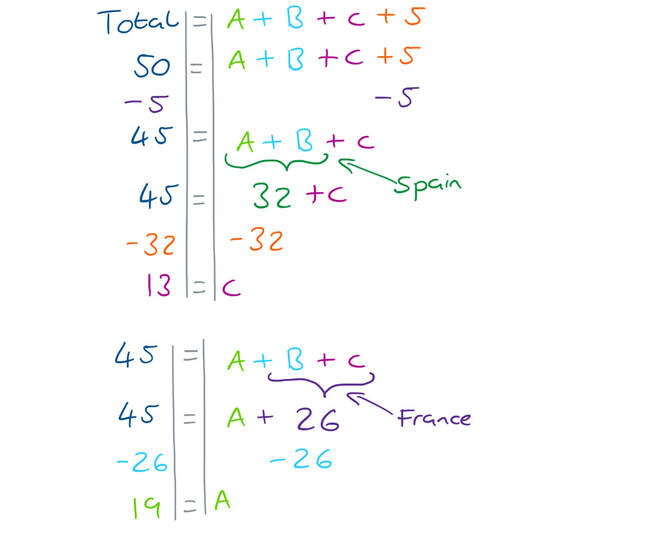
5) There are 50 individuals in a class. 32 of the individuals have been to Spain, 26 of the individuals have been to France and 5 of the individuals have not been to either.
a) Draw and compete and Venn diagram for the information above
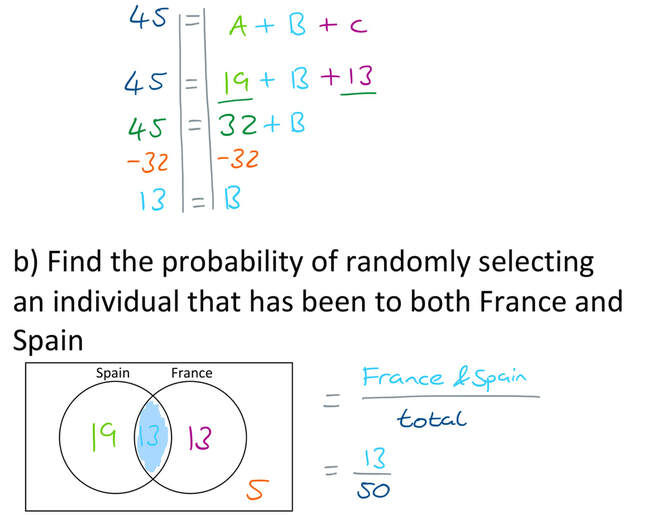
b) Find the probability of randomly selecting an individual that has been to both France and Spain
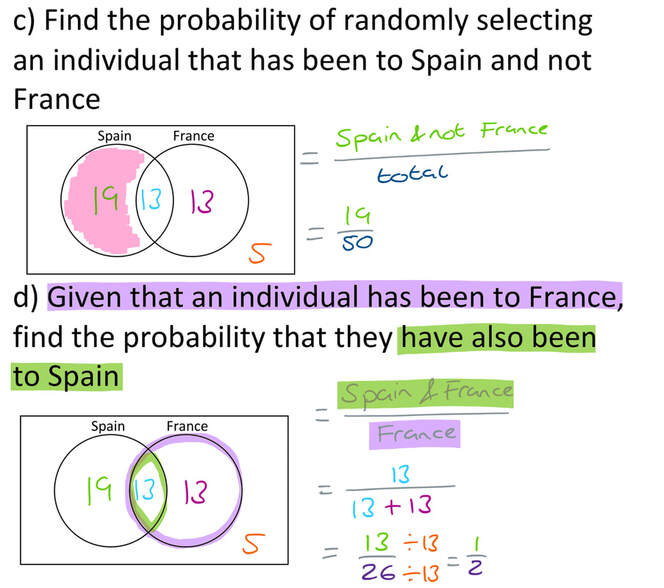
c) Find the probability of randomly selecting an individual that has been to Spain and not France
d) Given that an individual has been to France, find the probability that they have also been to Spain
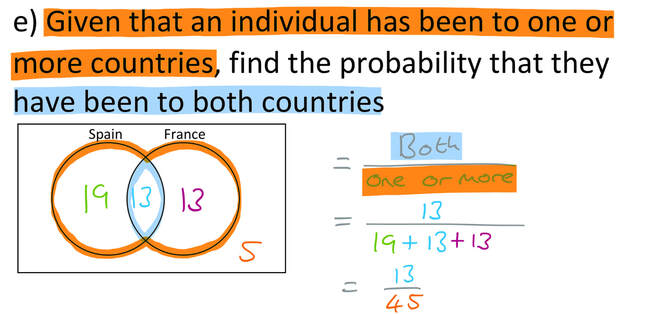
e) Given that an individual has been to one or more countries, find the probability that they have been to both countries
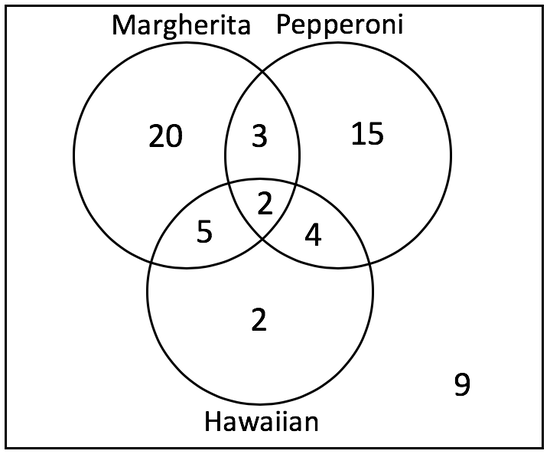
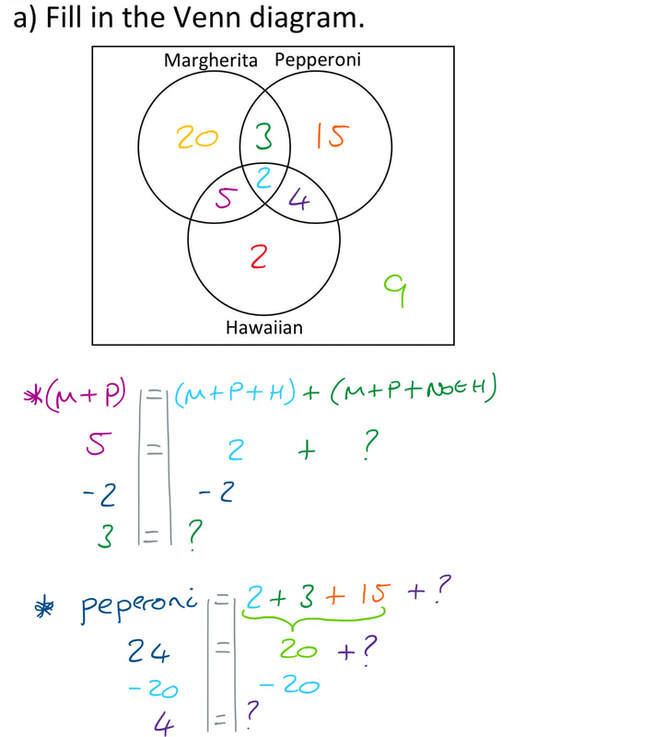
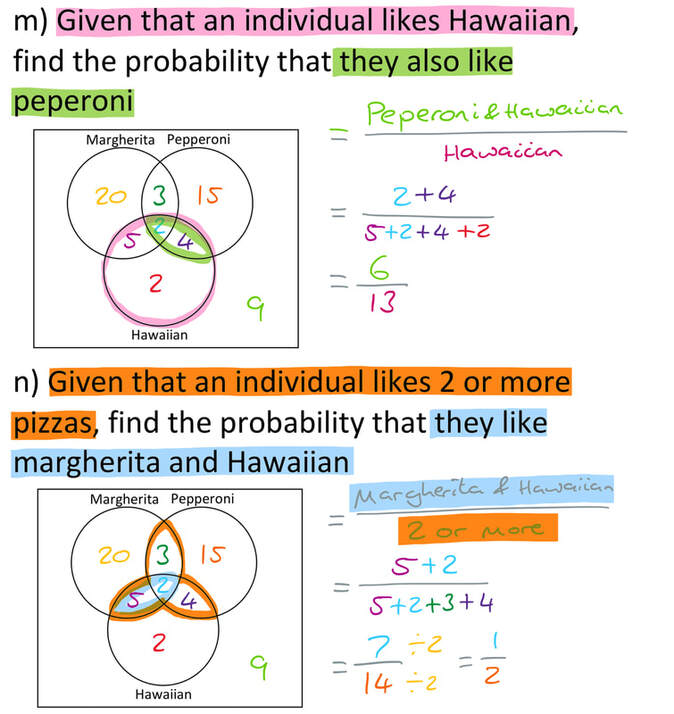
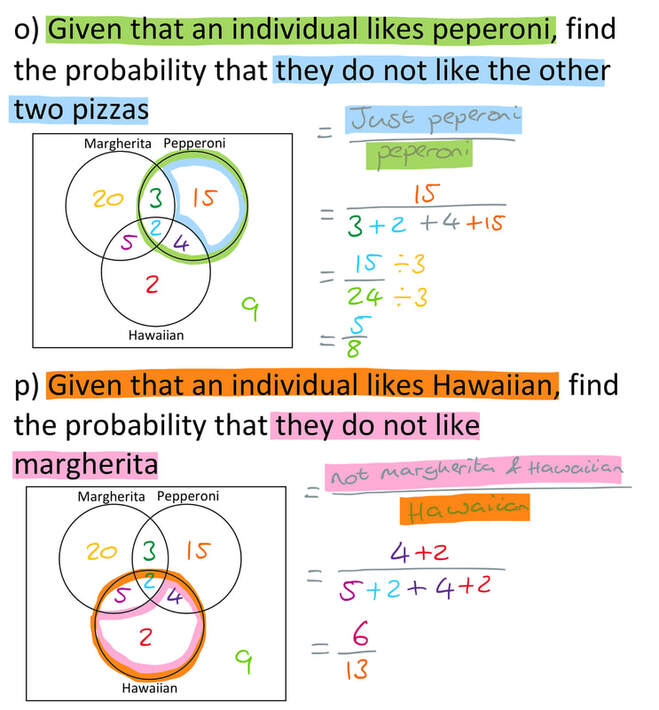
6) I ask 60 individuals what pizzas they like out of margarita, peperoni, Hawaiian. Some of the results are given below.
a) Fill in the Venn diagram.
c) A student is chosen at random. Find the probability that the student takes history and not geography
d) A student is chosen at random. Find the probability that the student takes history
e) Given that a student studies history, find the probability that they also study geography
f) Given that a student studies both subjects, find the probability that they study history
g) Given that a student does not study history, find the probability that they study geography
5) There are 50 individuals in a class. 32 of the individuals have been to Spain, 26 of the individuals have been to France and 5 of the individuals have not been to either.
a) Draw and compete and Venn diagram for the information above
b) Find the probability of randomly selecting an individual that has been to both France and Spain
c) Find the probability of randomly selecting an individual that has been to Spain and not France
d) Given that an individual has been to France, find the probability that they have also been to Spain
e) Given that an individual has been to one or more countries, find the probability that they have been to both countries
6) I ask 60 individuals what pizzas they like out of margarita, peperoni, Hawaiian. Some of the results are given below.
- 2 people liked all 3
- 15 people just liked peperoni
- 5 people like margherita and peperoni
- 24 people liked peperoni
- 9 people did not like any of the pizzas
- 7 people like margherita and Hawaiian
- 13 people liked Hawaiian
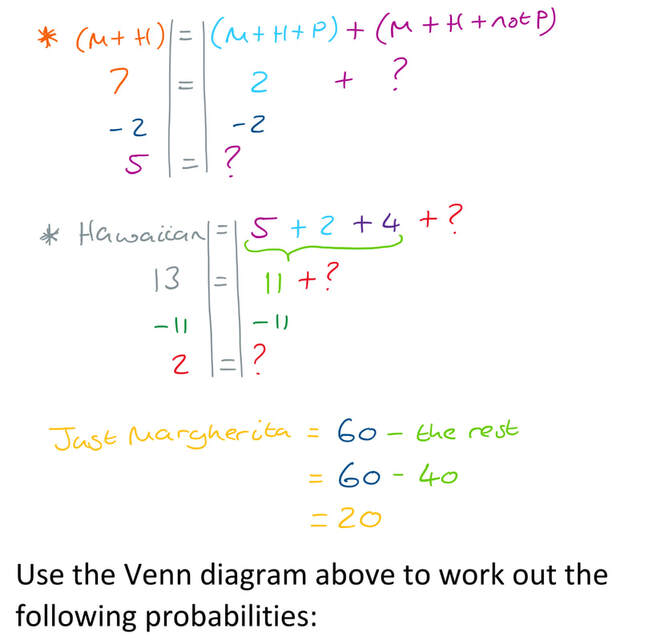
a) Fill in the Venn diagram.
Use the Venn diagram above to work out the following probabilities:
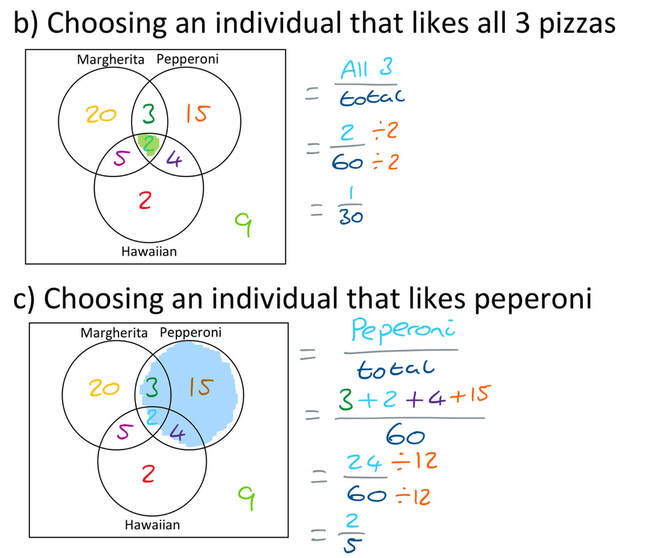
b) Choosing an individual that likes all 3 pizzas
c) Choosing an individual that likes peperoni
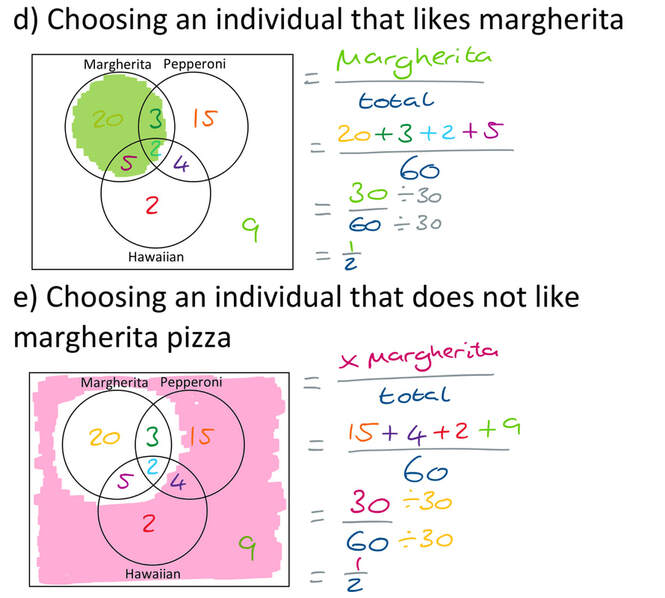
d) Choosing an individual that likes margherita
e) Choosing an individual that does not like margherita pizza
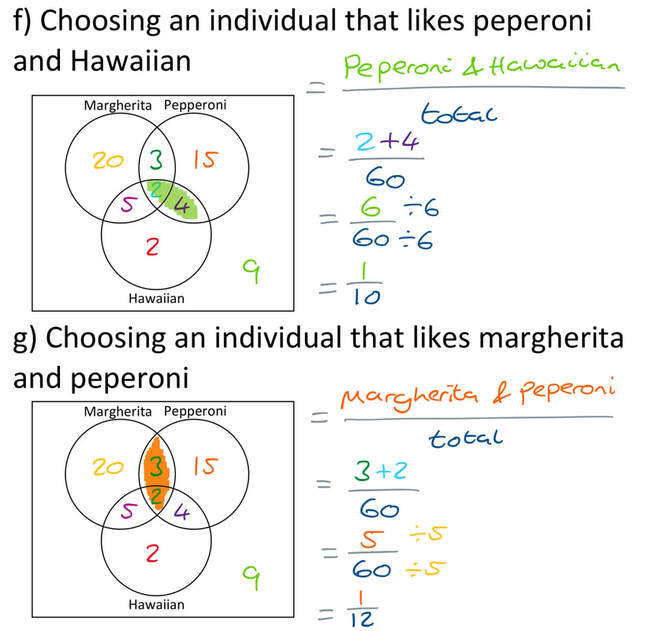
f) Choosing an individual that likes peperoni and Hawaiian
g) Choosing an individual that likes margherita and peperoni
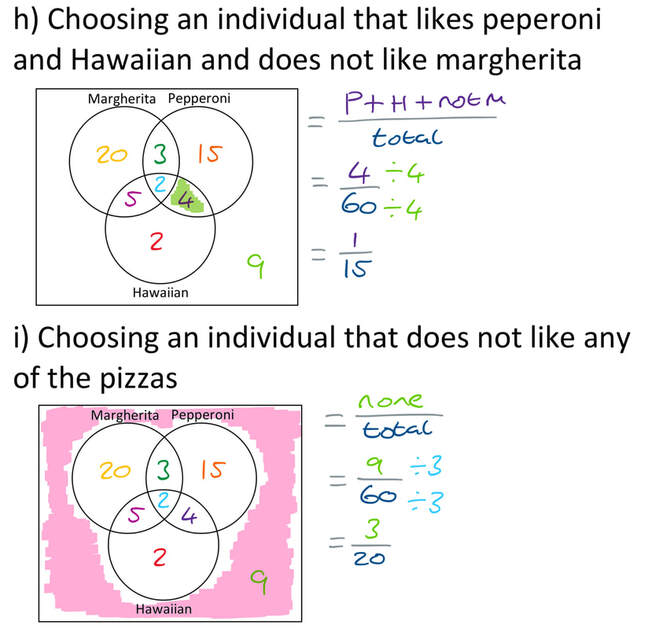
h) Choosing an individual that likes peperoni and Hawaiian and does not like margherita
i) Choosing an individual that does not like any of the pizzas
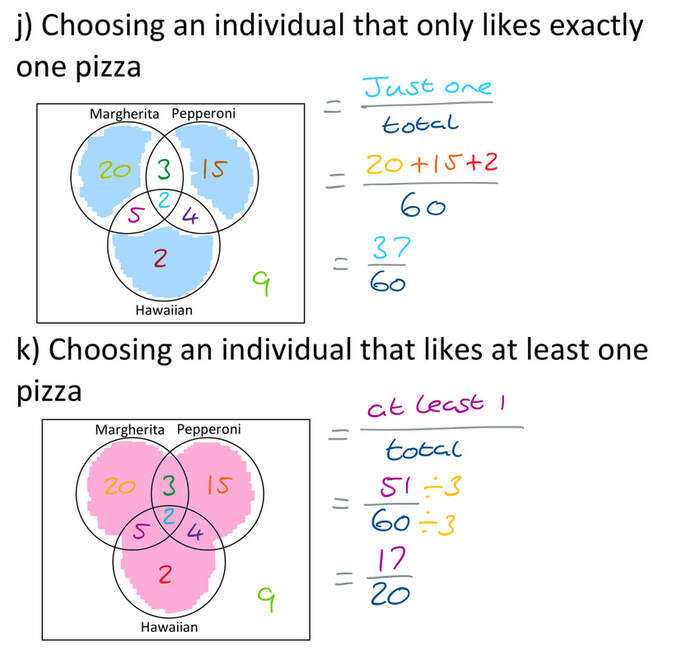
j) Choosing an individual that only likes exactly one pizza
k) Choosing an individual that likes at least one pizza
The following questions are about conditional probability.
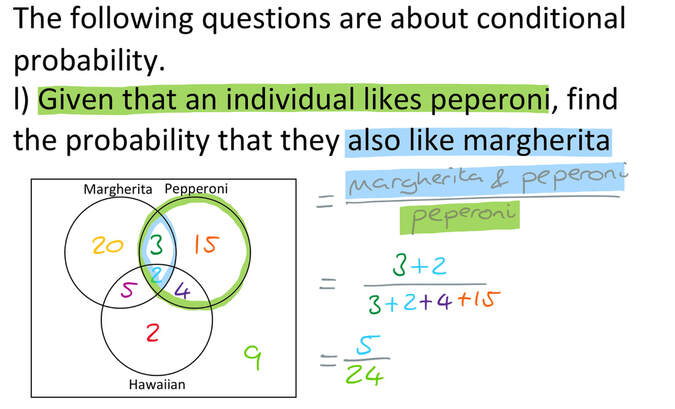
l) Given that an individual likes peperoni, find the probability that they also like margherita
m) Given that an individual likes Hawaiian, find the probability that they also like peperoni
n) Given that an individual likes 2 or more pizzas, find the probability that they like margherita and Hawaiian
o) Given that an individual likes peperoni, find the probability that they do not like the other two pizzas
p) Given that an individual likes Hawaiian, find the probability that they do not like margherita
b) Choosing an individual that likes all 3 pizzas
c) Choosing an individual that likes peperoni
d) Choosing an individual that likes margherita
e) Choosing an individual that does not like margherita pizza
f) Choosing an individual that likes peperoni and Hawaiian
g) Choosing an individual that likes margherita and peperoni
h) Choosing an individual that likes peperoni and Hawaiian and does not like margherita
i) Choosing an individual that does not like any of the pizzas
j) Choosing an individual that only likes exactly one pizza
k) Choosing an individual that likes at least one pizza
The following questions are about conditional probability.
l) Given that an individual likes peperoni, find the probability that they also like margherita
m) Given that an individual likes Hawaiian, find the probability that they also like peperoni
n) Given that an individual likes 2 or more pizzas, find the probability that they like margherita and Hawaiian
o) Given that an individual likes peperoni, find the probability that they do not like the other two pizzas
p) Given that an individual likes Hawaiian, find the probability that they do not like margherita