Back to Approximation Foundation Home
1.2 D) Estimating Calculations – Part 1
1.2 D) Estimating Calculations – Part 1
We often estimate calculations in the real world to roughly work stuff out. When we are estimating calculation, we generally round each of the numbers in the calculation to one significant figure. Also, when we are estimating a calculations, we use the sign ≈, which means “approximately equal to” rather than =, which means “equal to”.
Let’s have a few examples:
Let’s have a few examples:
Example 1
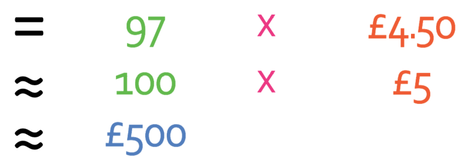
I am holding a concert. I have sold 97 tickets and each ticket cost £4.50.
a) Find an estimate for the amount of money that I have made from ticket sales by rounding each of the numbers to 1 significant figure.
b) Is the answer that you obtain an under or overestimate for the amount of money that I have brought in from ticket sales?
Part a in the questions asks us to estimate the amount of money made from ticket sales. The first step in finding this out is to work out what calculation I need to undertake to find the amount of money made from ticket sales. We are able to work this out by multiplying the number of tickets that are sold by the price for each ticket.
I am holding a concert. I have sold 97 tickets and each ticket cost £4.50.
a) Find an estimate for the amount of money that I have made from ticket sales by rounding each of the numbers to 1 significant figure.
b) Is the answer that you obtain an under or overestimate for the amount of money that I have brought in from ticket sales?
Part a in the questions asks us to estimate the amount of money made from ticket sales. The first step in finding this out is to work out what calculation I need to undertake to find the amount of money made from ticket sales. We are able to work this out by multiplying the number of tickets that are sold by the price for each ticket.
We are finding an estimate for the money made from selling tickets and we are obtaining this estimate by rounding each of the numbers above to 1 significant figure. The 97 rounds to 100 and the £4.50 rounds to £5 (see the video for a more detailed explanation as to how these numbers have been rounded). The calculation with the rounded numbers becomes:
Therefore, an estimate for the amount of money made from selling tickets is £500.
The second part of the question asks us to find out whether this is an under or overestimate. We find this out by thinking about the rounding that we undertook. We rounded both of the numbers up; the number of tickets sold was rounded up from 97 to 100 and the price of the ticket was rounded up from £4.50 to £5. Therefore, to find our estimate for the amount of money made from ticket sales, we multiplied two numbers that were larger than the actual numbers. This means that our estimate is an overestimate for the amount of money made from ticket sales.
Just to prove that our estimated value was an overestimate, I have worked out the actual amount that they would have taken from ticket sales, which was £436.50 (97 x £4.50). Our estimate was £500, which is above the actual amount, thus meaning that our estimate is an overestimate.
The second part of the question asks us to find out whether this is an under or overestimate. We find this out by thinking about the rounding that we undertook. We rounded both of the numbers up; the number of tickets sold was rounded up from 97 to 100 and the price of the ticket was rounded up from £4.50 to £5. Therefore, to find our estimate for the amount of money made from ticket sales, we multiplied two numbers that were larger than the actual numbers. This means that our estimate is an overestimate for the amount of money made from ticket sales.
Just to prove that our estimated value was an overestimate, I have worked out the actual amount that they would have taken from ticket sales, which was £436.50 (97 x £4.50). Our estimate was £500, which is above the actual amount, thus meaning that our estimate is an overestimate.
Multiplication – Under or Overestimate
We can only say that our estimate is an under or overestimate when we are multiplying if all of the numbers that we are multiplying are rounded in the same direction (such as all of the numbers are rounded up, or all of the numbers are rounded down).
In the above example, we rounded both of the numbers up, which meant that we were multiplying two numbers that were larger than their respective numbers in the actual multiplication calculation. This resulted in the estimate being an overestimate.
We can only say that our estimate is an under or overestimate when we are multiplying if all of the numbers that we are multiplying are rounded in the same direction (such as all of the numbers are rounded up, or all of the numbers are rounded down).
In the above example, we rounded both of the numbers up, which meant that we were multiplying two numbers that were larger than their respective numbers in the actual multiplication calculation. This resulted in the estimate being an overestimate.
If we were to round all of the numbers in a multiplication calculation down, our estimate would be an underestimate because we are multiplying numbers that are less than their respective numbers in the multiplication calculation.