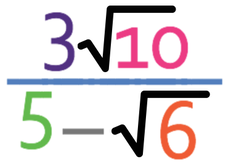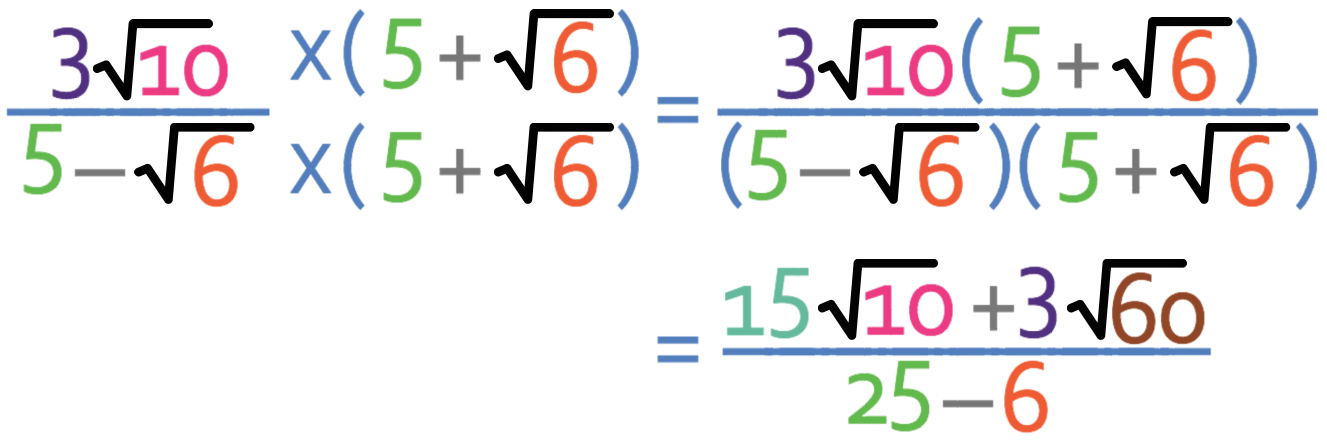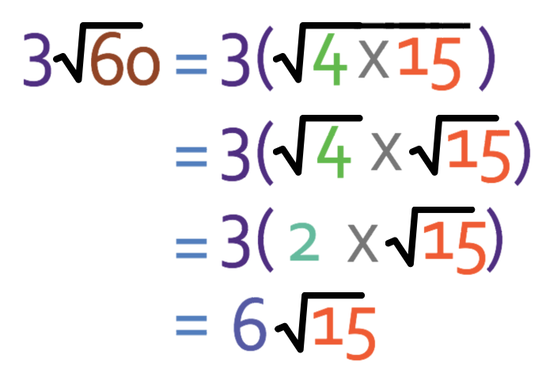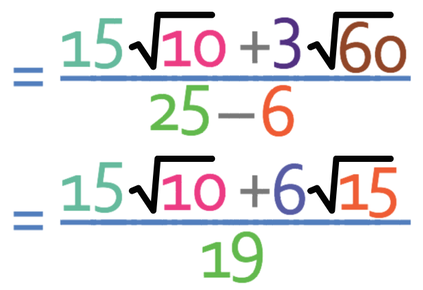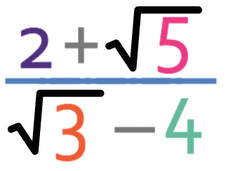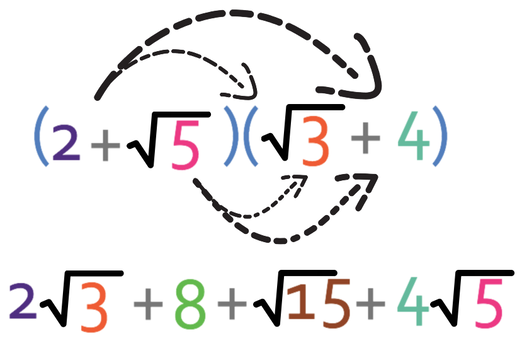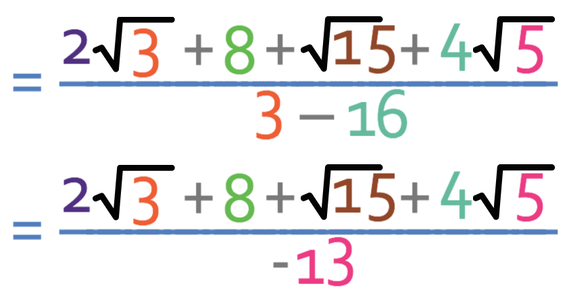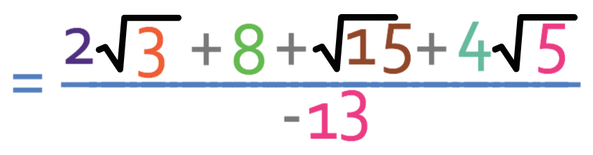Back to Edexcel Surds (H) Home
1.8 I) Rationalising Denominators – Part 3
1.8 I) Rationalising Denominators – Part 3
The content in this section builds on the content that was discussed in the previous section. Make sure that you have gone through the content in the previous section before working through the content in this section (click here to be taken through to the previous section).
Example 1
Rationalise the denominator for the fraction below.
Rationalise the denominator for the fraction below.
The denominator of the fraction is 5 – √6, which means that we are going to be multiplying the numerator and the denominator of the fraction by 5 + √6.
We now need to check if the answer can be simplified; we need to check all of the surds for square factors and check the numerator and the denominator for any common factors.
There are no square factors in 10, which means that √10 is in its simplest form. We now check √60 for square factors. 4 is a square number and a factor of 60, which means that the second surd can be simplified. The simplification process for the second term in the numerator is shown below:
There are no square factors in 10, which means that √10 is in its simplest form. We now check √60 for square factors. 4 is a square number and a factor of 60, which means that the second surd can be simplified. The simplification process for the second term in the numerator is shown below:
This means that the fraction becomes:
We now need to check whether there are any common factors in 15, 6 and 19. There are no common factors, which means that the fraction above is in its simplest form. Therefore, the answer to this question is:
Example 2
Rationalise the denominator for the fraction below.
Rationalise the denominator for the fraction below.
The denominator of the fraction is √3 – 4 and in order to rationalise this fraction we are going to be multiplying it by √3 + 4.
For the numerator, we are now multiplying out two brackets each with two terms in them. When we are multiplying out these double brackets, we need to make sure that we multiply all of the terms in the first bracket by all of the terms in the second bracket. The best way to ensure that we do this is to multiply out the brackets using FOIL (First, Outside, Inside and Last). The multiplication for the numerator is shown below.
The denominator is the difference of two squares and therefore quite straight forwards to multiply out. We will get: ((√3)2) – 42 = 3 – 16 = -13.
Finally, we need to check whether the fraction can be simplified.
We start by checking whether the surds can be simplified. We check whether the surds can be simplified by looking for square factors in the numbers inside each of the surds; there are no square factors in 3, 15 or 5, thus meaning that all of the surds are in their simplest form.
The next step is to check if there are any common factors in the numerator and the denominator, which there aren’t. This means that the fraction is in its simplest form. The final answer is given below:
We start by checking whether the surds can be simplified. We check whether the surds can be simplified by looking for square factors in the numbers inside each of the surds; there are no square factors in 3, 15 or 5, thus meaning that all of the surds are in their simplest form.
The next step is to check if there are any common factors in the numerator and the denominator, which there aren’t. This means that the fraction is in its simplest form. The final answer is given below: